I extend my sincere gratitude and acknowledgment to Dr. Safyan Majid, from the Institute of Business & Management (IB&M), UET Lahore, for his invaluable assistance and insights in shaping this knowledge. His expertise in Financial Management has greatly enriched the content, making it more relevant to contemporary finance practices. This acknowledgment also highlights his continuous contributions to promoting awareness and fostering meaningful discourse on Corporate Social Responsibility (CSR), benefiting both the student and professional community
For detail pl review the post
- https://solbiztech.com/blog/sbt-blog-1/the-role-of-financial-management-objectives-and-functions-13
- https://solbiztech.com/blog/sbt-blog-1/value-creation-agency-problem-and-corporate-social-responsibilities-in-financial-management-14
- https://solbiztech.com/blog/sbt-blog-1/structure-of-corporate-governance-in-financial-management-16
1. Introduction
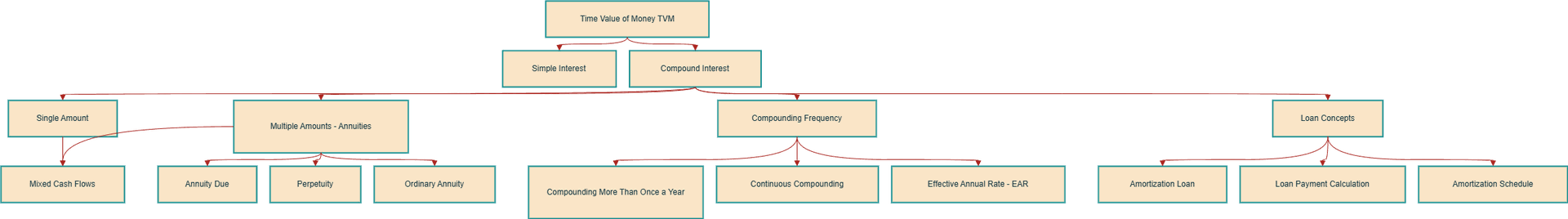
The Time Value of Money (TVM) is a fundamental concept in finance that recognizes the principle that a rupee today is worth more than a rupee in the future. This is due to its earning potential and the effects of interest.
Understanding TVM helps in making informed decisions about investments, loans, savings, and financial planning. This article covers:
- Simple vs. Compound Interest
- Single Amount & Annuities
- Compounding Frequency & Continuous Compounding
- Effective Annual Rate (EAR)
- Loan Amortization & Mixed Cash Flows
- Business Cases Where TVM is Applied
2. Business Cases for Time Value of Money
Understanding the business cases where TVM is applied helps in making better financial decisions. Below are some key financial management areas where TVM plays a crucial role:
2.1 Investment Decision Making
- Investors use TVM to compare different investment options and evaluate whether future cash flows from an investment justify the present expenditure.
- Example: Deciding whether to invest in stocks, bonds, or mutual funds by calculating their Net Present Value (NPV) and Internal Rate of Return (IRR).
2.2 Loan and Mortgage Planning
- Financial institutions and individuals use TVM to determine loan repayment schedules and monthly installments.
- Example: A borrower deciding between a fixed-rate mortgage vs. adjustable-rate mortgage using present value calculations.
2.3 Retirement and Pension Planning
- TVM is used to estimate how much an individual should invest today to meet future retirement goals.
- Example: Calculating how much needs to be saved monthly in a retirement fund to ensure financial security post-retirement.
2.4 Business Valuation and Mergers
- TVM is used in business valuation models to determine the worth of a company by discounting future cash flows.
- Example: A company considering acquiring another company evaluates the discounted future earnings to determine the offer price.
2.5 Capital Budgeting for Projects
- Businesses use TVM to analyze whether long-term projects are financially viable by discounting future project cash flows.
- Example: A company deciding whether to build a new factory by comparing the Present Value of Costs vs. Future Expected Revenues.
2.6 Lease vs. Buy Decisions
- Organizations use TVM to evaluate whether to lease equipment or buy it outright based on the present value of future payments.
- Example: A manufacturing firm comparing the cost of leasing vs. buying machinery over a 5-year period.
2.7 Bond Pricing and Fixed-Income Securities
- TVM is crucial in determining the present and future value of bonds and fixed-income securities.
- Example: Investors calculating the Yield to Maturity (YTM) of a bond to determine whether it is worth investing in.
2.8 Insurance and Annuities Pricing
- Insurance companies use TVM to determine premium amounts based on expected future payouts.
- Example: Calculating life insurance premiums and annuity payouts based on expected future claims and interest rates.
3. Simple vs. Compound Interest
3.1 Simple Interest
Definition: Simple interest is the interest calculated only on the initial principal amount for a specific period. It does not compound over time.
Simple Interest is calculated only on the initial principal:
SI = PV * i * n
FV = PV + SI or FV = PV + (PV * i * n)
PV = FV / [1 + (i * n)]
Example Calculation:
- PV = 1,000, i = 10%, n = 5
- FV = 1,000 + (1,000 * 0.10 * 5) = 1,500
- PV = 1,500 / (1 + 0.10 * 5) = 1,000
3.2 Compound Interest
Definition: Compound interest is the interest calculated on both the initial principal and the accumulated interest from previous periods. This allows for exponential growth over time.
3.2.1 Single Amount
Compound Interest is calculated on both the principal and previously accumulated interest:
FV = PV * (1 + i)^n or FV = PV * FVIF
PV = FV / (1 + i)^n or PV = FV * PVIF
Example Calculation:
- PV = 1,000, i = 10%, n = 5
- FV = 1,000 * (1.10)^5 = 1,610.51
- PV = 1,610.51 / (1.10)^5 = 1,000
3.2.2. Multiple Amounts (Annuities & Perpetuities)
3.2.2.1 Ordinary Annuity
Definition: An ordinary annuity is a series of equal cash flows occurring at the end of each period. It is commonly used in loan repayments and investment calculations.
FVA = R * [(1 + i)^n - 1] / i or FVA = R * FVIFA
PVA = R * (1 - (1 + i)^-n) / i or PVA = R * PVIFA or PVA = R * [1 - [(1 / (1+i)^n )]] / i
Example Calculation:
- R = 500, i = 8%, n = 5
- FVA = 500 * ((1.08)^5 - 1) / 0.08 = 2,926.56
- PVA = 500 * (1 - (1.08)^-5) / 0.08 = 1,996.46
3.2.2.2 Annuity Due
Definition: An annuity due is a series of equal payments made at the beginning of each period rather than the end. This results in greater accumulated value over time due to earlier compounding.
FVAD = R * (1 + i) * ((1 + i)^n - 1) / i or FVAD = R (1+i) * FVIFA
PVAD = R * (1 + i) * (1 - (1 + i)^-n) / i or PVAD = R (1+i) * FVIFA
Example Calculation:
- R = 500, i = 8%, n = 5
- FVAD = 500 * (1.08) * ((1.08)^5 - 1) / 0.08 = 3,160.68
- PVAD = 500 * (1.08) * (1 - (1.08)^-5) / 0.08 = 2,156.18
3.2.2.3 Perpetuity
Definition: A perpetuity is a type of annuity that provides continuous payments indefinitely. Since there is no end, its present value is calculated using a simple formula based on the periodic payment and the interest rate.
PVA = R / i
Example Calculation:
- R = 500, i = 8%
- PVA = 500 / 0.08 = 6,250
4. Compounding Frequency & EAR
4.1 Compounding More Than Once a Year
Definition: When interest is compounded more than once a year, the total accumulated interest increases due to more frequent calculations.
FV = PV * (1 + i/m)^(m * n)
PV = FV / (1 + i/m)^(m * n)
Example Calculation:
- PV = 1,000, i = 10%, m = 4 (quarterly), n = 3 years
- FV = 1,000 * (1 + 0.10/4)^(4*3) = 1,344.89
4.2 Continuous Compounding
Definition: Continuous compounding assumes that interest is calculated and added to the principal an infinite number of times per year, leading to maximum growth potential.
Example Calculation:
- PV = 1,000, i = 10%, n = 3 years
- FV = 1,000 * e^(0.10 * 3) = 1,349.86
FV = PV * e^(i * n)
PV = FV * e^(-i * n)
4.3 Effective Annual Rate (EAR)
Definition: The Effective Annual Rate (EAR) is the true interest rate an investor or borrower will earn or pay annually, considering the effects of compounding. It allows for fair comparisons between different interest rate structures.
EAR = [1 + (i/m)]^m - 1
Example Calculation:
- Nominal Rate (i) = 10%, Compounded Quarterly (m = 4)
- EAR = (1 + 0.10/4)^4 - 1 = 10.38%
5. Loan Amortization & Payment Schedules
5.1 Loan Amortization Formula
Definition: Loan amortization is the process of paying off a loan over time through regular payments. Each installment consists of both interest and principal repayment.
Hint: Same Ordinary Annuity PVA = R * PVIFA derived: R = PVA /PVIFA PVA = R * (1 - (1 + i)^-n) / i derived: R = PVA * (i / (1 - (1 + i)^-n))
Example Calculation:
- P = 10,000, i = 10%, n = 5
- R = 10,000 * (0.10 / (1 - (1.10)^-5)) = 2,637.97
5.2 Amortization Schedule
| Year | Installment Payment (R) | Interest (i * Beg. Balance) | Principal (Payment - Interest) | Amount Owing at Year End |
|---|---|---|---|---|
| 0 | - | - | - | 10,000 |
| 1 | 2,637.97 | 1,000.00 | 1,637.97 | 8,362.03 |
| 2 | 2,637.97 | 836.20 | 1,801.77 | 6,560.26 |
| 3 | 2,637.97 | 656.03 | 1,981.94 | 4,578.32 |
| 4 | 2,637.97 | 457.83 | 2,180.14 | 2,398.18 |
| 5 | 2,637.97 | 239.82 | 2,398.15 | 0 |
| Total | 13,189.85 | 4,189.88 | 10,000.00 | - |
7. Conclusion: TVM Methods and Use Cases
| Category | TVM Method | Future Value (FV) Formula | Present Value (PV) Formula | Definition | Use Case |
|---|---|---|---|---|---|
| Simple Interest | Basic Calculation | FV = PV * (1 + i * n) | PV = FV / (1 + i * n) | Interest earned on the original principal only over time. | Used in simple loans or savings with fixed interest, e.g., bank fixed deposits. |
| Single Amount | Compound Interest | FV = PV * (1 + i)^n | PV = FV / (1 + i)^n | Interest is calculated on both the initial principal and accumulated interest. | Used in investments where interest compounds, e.g., mutual funds. |
| Multiple Amounts (Annuities) | Ordinary Annuity | FVA = R * ((1 + i)^n - 1) / i | PVA = R * (1 - (1 + i)^-n) / i | A series of equal payments at regular intervals, paid at the end of each period. | Used for retirement savings, loan repayments, etc. |
| Multiple Amounts (Annuities) | Annuity Due | FVAD = R * (1 + i) * ((1 + i)^n - 1) / i | PVAD = R * (1 + i) * (1 - (1 + i)^-n) / i | A series of equal payments at regular intervals, paid at the beginning of each period. | Used in lease agreements and rent payments. |
| Multiple Amounts (Annuities) | Perpetuity | - | PVA = R / i | A constant stream of cash flows that continues indefinitely. | Used for valuing perpetual bonds and endowments. |
| Multiple Amounts (Annuities) | Mixed Cash Flows | FV = SUM(FV_i) | PV = SUM(FV_i / (1 + i)^n) | A combination of single and multiple cash flows occurring at different times. | Used in capital budgeting and project finance. |
| Compounding Frequency | More Than Once a Year | FV = PV * (1 + i/m)^(m * t) | PV = FV / (1 + i/m)^(m * t) | Interest is compounded more frequently than once per year. | Used in mortgages, credit cards, and savings accounts. |
| Compounding Frequency | Continuous Compounding | FV = PV * EXP(i * t) | PV = FV * EXP(-i * t) | Compounding occurs an infinite number of times per year, leading to exponential growth. | Used in high-frequency financial models and advanced banking applications. |
| Compounding Frequency | Effective Annual Rate (EAR) | EAR = (1 + i/m)^m - 1 | - | An interest rate expressed as if it were compounded once per year. | Used to compare different investment or loan options. |
| Loan Concepts | Amortization Loan | - | R = P * (i / (1 - (1 + i)^-n)) | A loan where the borrower makes periodic payments of principal and interest. | Used in home loans, car loans, and personal financing. |
| Loan Concepts | Loan Payment Calculation | - | Total Payment = Interest + Principal | The periodic payment amount required to repay a loan over time. | Used to determine EMI for loans and credit repayments. |
| Loan Concepts | Amortization Schedule | - | Shows how balance reduces over time | A schedule showing how loan payments are split between interest and principal. | Used to track loan balance over time, including interest and principal breakdown. |
Author:
Mohsin Yaseen
On behalf of SolBizTech Team
https://www.linkedin.com/in/rmyasin